5.2 Binomial Option Pricing:
N-Periods
|
|
n this topic, we apply the risk-neutral valuation technique to value a European call option that lasts for n-periods. The presentation is based upon the work of Cox, Ross, and Rubinstein (1979).
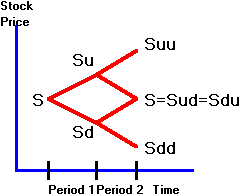
The tree for the stock in the two-period problem is shown in
Figure 5.1.
Figure 5.1
Binomial Tree:
2-Period
In this case, we can write the call option value with respect
to risk-neutral probabilities p as:
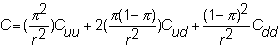
This two-period form can be rearranged into the general N-period
binomial option pricing model by summing over the 2n
possible paths that the underlying stock price can take.
To take a closer look at the derivation of how the N-period
model generalizes the two-period model, you can read topic 5.3, Binomial
Option Pricing Model: N-Period
Derivation.
The n-period
binomial option pricing model is expressed as:
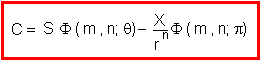
Here, p
is the risk-neutral probability of a realized uptick u,
and q = pu/r, which has the property that 0 < q < 1 because q
= u(r - d)/(r(u - d))
and u > r. We can
interpret q
as a "risk-neutral terminal value-weighted probability." F is
the probability of at least m upticks occurring from n ticks computed relative
to risk-neutral probabilities p, and
risk-neutral value-weighted probabilities q.
This model includes two binomial probability distribution
terms as a function of n, the number
of periods, and m, which is the
smallest number of upticks required for the call option to finish
"in-the-money."
The first term is the present value of the expected revenue
from exercising an in-the-money option. This
term is not simply equal to S times
the probability of the call being in-the-money, because the expected revenue
depends on the terminal stock price associated with each path.
The critical information associated with a path and its terminal value is
the number of upticks realized plus the constants u
and r along the path. Therefore,
we can calculate the expected revenue from the current stock price weighted by
the risk-neutral terminal value-weighted probability.
The second term is the (risk-neutral) probability that the
call option will be in-the-money multiplied by the exercise price and discounted
by the risk-free rate. Therefore,
it is the present value of the expected exercise cost.
If you want to see the derivation of how the N-period
model generalizes the two-period model, see
topic 5.3, Binomial Option Pricing
Model: N-Period
Derivation, otherwise you can skip to the limiting behavior of the binomial
option pricing model, as periods become smaller and smaller, in
topic 5.4, Binomial Option Pricing:
Limiting Results).
 office (412)
9679367
office (412)
9679367